Abstract:
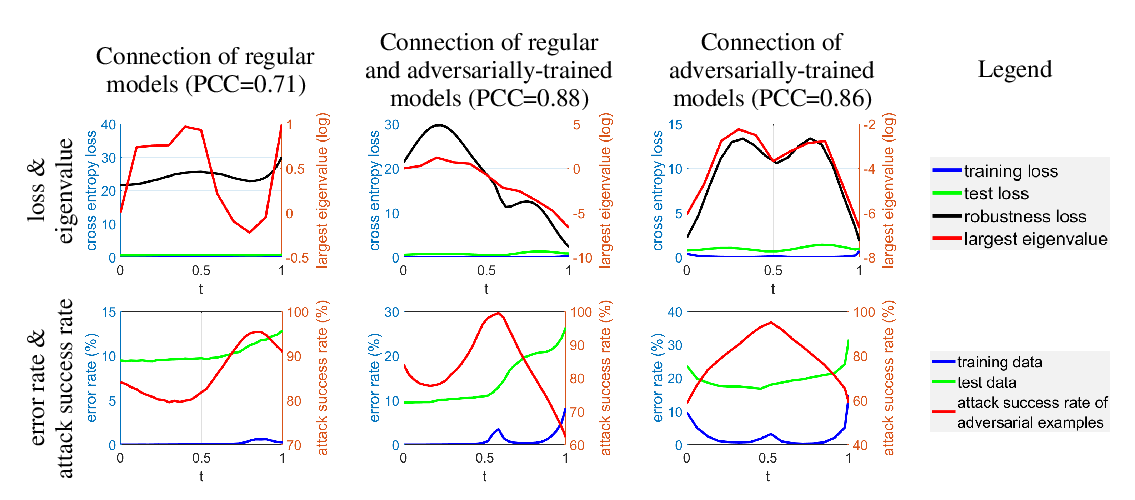
Neural ordinary differential equations (ODEs) have been attracting increasing attention in various research domains recently. There have been some works studying optimization issues and approximation capabilities of neural ODEs, but their robustness is still yet unclear. In this work, we fill this important gap by exploring robustness properties of neural ODEs both empirically and theoretically. We first present an empirical study on the robustness of the neural ODE-based networks (ODENets) by exposing them to inputs with various types of perturbations and subsequently investigating the changes of the corresponding outputs. In contrast to conventional convolutional neural networks (CNNs), we find that the ODENets are more robust against both random Gaussian perturbations and adversarial attack examples. We then provide an insightful understanding of this phenomenon by exploiting a certain desirable property of the flow of a continuous-time ODE, namely that integral curves are non-intersecting. Our work suggests that, due to their intrinsic robustness, it is promising to use neural ODEs as a basic block for building robust deep network models. To further enhance the robustness of vanilla neural ODEs, we propose the time-invariant steady neural ODE (TisODE), which regularizes the flow on perturbed data via the time-invariant property and the imposition of a steady-state constraint. We show that the TisODE method outperforms vanilla neural ODEs and also can work in conjunction with other state-of-the-art architectural methods to build more robust deep networks.